This piece is a set of supplementary notes on General Relativity, and it may also serve as a extended reading for tensor analysis.
This note extends the familiar rank-2 split,
$$
T_{ab}=T_{(ab)}+T_{[ab]},
$$
to rank-$n$ tensors via the natural action of the symmetric group $S_n$ that permutes tensor factors of $V^{\otimes n}$.
Invariant subspaces ↔ irreps of $S_n$.
Symmetry types of indices correspond to irreducible $S_n$-modules (Specht modules) labeled by Young diagrams $\lambda\vdash n$.
Projectors.
Central (character) projectors select the $\lambda$-isotypic component; Young symmetrizers further isolate a single copy with the prescribed row-symmetry / column-antisymmetry.
Schur–Weyl viewpoint.
Commuting actions of $S_n$ and $\mathrm{GL}(V)$ yield
$$
V^{\otimes n}\cong\bigoplus_{\lambda\vdash n,\ \ell(\lambda)\le \dim V} S_\lambda(V)\otimes \mathrm{Specht}_\lambda,
$$
explaining multiplicities and the viability condition $\ell(\lambda)\le \dim V$.
How to use: pick a Young diagram $\lambda$, apply the one-line character projector to any $T\in V^{\otimes n}$ to get its $\lambda$-component, and optionally use a Young symmetrizer to extract one mixed-symmetry copy. The rank-2 case appears as $\lambda=(2)$ (totally symmetric) and $\lambda=(1,1)$ (totally antisymmetric).
分类： General Relativity
Lecture notes 14.11.20
This is my draft for a 20min introduction to gravitational wave for sophomore students, presented on Prof.Fan’s Theoretical Mechanics course. A rearranged LaTeX version may be uploaded later.
This article using Schwarzschild metric as an example to demonstrate the calculation of Christoffel symbols and their contraction with given metric
Codes used in this post has been published on Wolfram cloud and is completely open source.. Loading online notebook may take a while, take a break for ur health 🙂
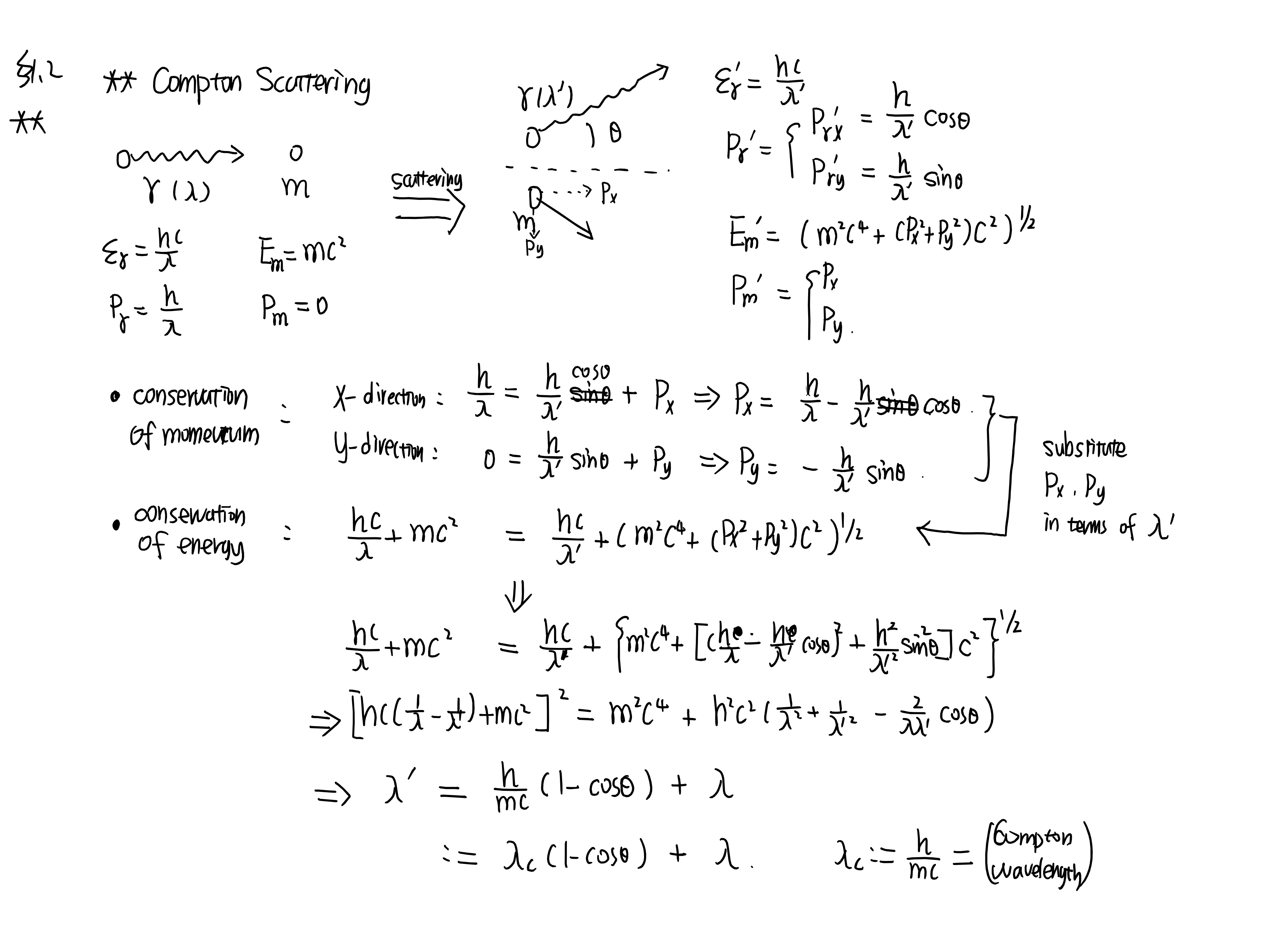
1.2 Photon
solution of Compton scattering may also serve as an exercise of relativity
This note can be use as an example of tensor analysis
This article shows the derivation of Newtonian tidal effect from Newton’s equation of motion and gravity, with the notation of tensor analysis and Einstein summation convention. This may serve as an example for beginners in tensor analysis to check their comprehension, meanwhile as a baby version or lead-in to geodesic deviation of general relativity.
Newton’s second law (componentwise)
$$
\frac{d^{2} x^{i}}{d t^{2}} \equiv a^{i} \equiv \frac{F^{i}}{m}
$$
Newtonian equation of gravitation
$$
\frac{F^{i}}{m}=-\eta^{i j} \partial_{j} \phi
$$
combine two equations give:
$$
\frac{d^{2} x^{i}}{d t^{2}}=-\eta i j\left[\partial_{j} \phi\right]_{\vec{x}}
$$
where the subscription $\vec{x}$ denotes the derivative is operated at position $\vec{x}$ similarly we have at $\vec{x}+\vec{n}$ :
$$
\frac{d^{2}\left(x^{i}+n^{i}\right)}{d t^{2}}=-\eta^{i j}\left[\partial_{j} \phi\right]_{\vec{x}+\vec{n}}
$$
make subtraction give:
$$
\frac{d^{2} n^{i}}{d t^{2}}=-\eta^{i j}\left(\left[\partial_{j} \phi\right]{\vec{x}+\vec{n}}-\left[\partial{j} \phi\right]_{\vec{x}}\right)
$$
given $\vec{n}$ is infinitesimal, we have RHS:
$$
\text { RHS }=-\eta^{i j} n^{k}\left[\partial_{k}\left(\partial_{j} \phi\right)\right]_{\vec{x}}
$$
set Cartesian coordinates originated from centre of earth orient the $z$-axis so that is consists with $\vec{x}$ (position vector) then $\vec{X}=\left(X^{1}, X^{2}, X^{3}\right)=(0,0, z)$.
gravitational potential is given by:
$$
\phi=-\frac{G M}{r}=-\frac{G M}{\left(x^{2}+y^{2}+z^{2}\right)^{1 / 2}}
$$
and thus
$$
\begin{aligned}
\partial_{j} \phi & =\frac{\partial \phi}{\partial x^{j}} \\
& =-\frac{d \phi}{d r} \frac{\partial r}{\partial x^{j}} \\
& =\frac{G M}{r^{3}} x^{j}
\end{aligned}
$$
and
$$
\begin{aligned}
\partial_{k} \partial_{j} \phi & =\frac{\partial}{\partial x^{k}}\left(\frac{G M}{r^{3}} x^{j}\right) \\
& =\frac{\partial}{\partial x^{k}}\left(\frac{G M}{r^{3}}\right) x^{j}+\delta_{k}^{j} \frac{G M}{r^{3}} \\
& =-\frac{3 G M}{r^{4}} x^{j}+\delta_{k}^{j} \frac{G M}{r^{3}}
\end{aligned}
$$
thus.
$$
\begin{aligned}
& \frac{d^{2}}{d t^{2}} n^{x}=-\frac{G M}{r^{3}} n^{x} \\
& \frac{d^{2}}{d t^{2}} n^{y}=-\frac{G M}{r^{3}} n^{y} \\
& \frac{d^{2}}{d t^{2}} n^{z}=\frac{2 G M}{r^{3}} n^{z}
\end{aligned}
$$